OpenIntroOrg Casio fx-9750GII - Binomial Formula (Distribution, Including Cumulative Distribution)

Compute a binomial formula (distribution) and binomial cumulative distribution on a Casio 9750 graphing calculator ( http://amzn.to/1AVxr78).

For more free statistics resources, visit http://www.openintro.org.

Also, check out our free intro statistics textbooks at http://www.openintro.org/stat/textboo....

- - -

Approximate script:

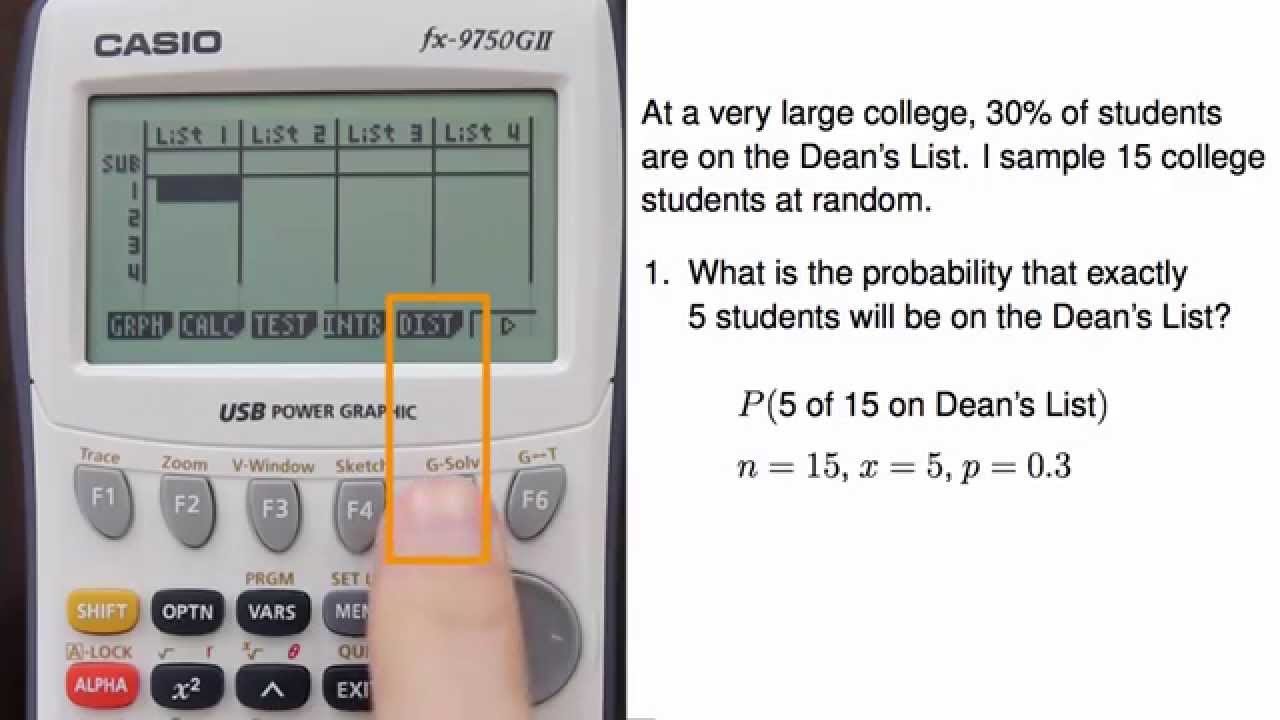
I'm going to cover 3 binomial distribution problems using a Casio 9750 graphing calculator. Suppose at a very large college, 30% of students are on the Dean's List. Then suppose I sample 15 college students at random. What is the probability that exactly 5 of the students will be on the Dean's List?

The first problem requires the binomial formula since I want to know what is the probability that exactly 5 of the 15 students will be on the Dean's List. In this example, the number of trials is n = 15, the number of students who satisfy the condition is x = 10, and the probability a random student satisfies the condition is 0.3.

I can compute this result on the Casio calculator by first going to the Stat section using Menu and then hitting 2. Then I can hit F5 for Distributions, F5 again for Binomial distribution, and F1 for Binomial probability distribution. Next, I enter the details into the calculator. Here it's showing the list view, and I'll need to switch to the variable view by hitting F2. Next I can enter x, which is 5, the number of trials, which is 15, and the probability 0.3. Hitting execute gives the binomial probability: 0.2061.

The second question is, what is the probability that fewer than 4 will be on the Dean's List?

I care about the probability that fewer than 4 of the 15 be on the Dean's List. That is, it can be 0, 1, 2, or 3 students. I could compute the binomial probabilities for each of these scenarios and then add them together, but that would take awhile. Instead, I should use the Binomial cumulative distribution function, which gives the left tail of a binomial distribution. To use it, I'll need to again identify our parameters. As before, the number of trials is 15. For a cumulative distribution, I identify the cutoff for the left tail, which in this case is 3, since I'm looking for fewer than 4. Then the probability is the same as before: 0.3.

I'll exit out to the Stat section. As before, I can go into Distribution, then Binomial. To get the Binomial cumulative distribution, I choose F2 for Bcd. Now I can again enter in the context: x is 3, number of trials is 15, and probability is 0.3. Hitting execute gives the answer: 0.2969.

The last question is, what is the probability that 7 or more will be on the Dean's List?

I want to compute the probability that 7 or more of the 15 students will be on the Dean's List. This is the right tail, and while the calculator won't give me a right tail, I can compute the complement, which is the left tail, and then subtract that from 1 to get the right tail. So as before, I determine x, which in this case will be 6, since I want 7 to be included in the upper tail, the number of trials as 15, and the probability as 0.3.

Exiting back to the Binomial cumulative distribution, I can change the 3 to a 6 and hit execute: 0.8689. And again, this is the lower tail. To get the upper tail, I take 1 minus 0.8689, which is 0.1311.

If you liked this video, give it a thumbs up below and subscribe. Thanks for watching!

Casio fx-9750GII - Binomial Formula (Distribution, Including Cumulative Distribution)
859 Likes	859 Dislikes
62,309 views views	5,580 followers
Education	Upload TimePublished on 12 May 2015